In trigonometry, sin, cos, and tan are the basic trigonometric ratios used to study the relationship between the angles and sides of a triangle (especially of a right-angled triangle). Pythagoras worked on the relationship between the sides of a right triangle through the Pythagorean theorem while Hipparcus worked on establishing the relationship between the sides and angles of a right triangle using the concepts of trigonometry. Sin, cos, and tan formulas in trigonometry are used to find the missing sides or angles of a right-angled triangle.
Let’s understand the sin, cos, and tan in trigonometry using formulas and examples.
1. What is Sin Cos Tan in Trigonometry? 2. Sin Cos Tan Formula 3. Sin Cos Tan Table 4. Tips to Remember Sin Cos Tan Table 5. Sin Cos Tan on Unit Circle 6. Application of Sin Cos Tan in Real Life 7. FAQs on sin cos tan
Sin, cos, and tan are the three primary trigonometric ratios, namely, sine, cosine, and tangent respectively, where each of which gives the ratio of two sides of a right-angled triangle. We know that the longest side of a right-angled triangle is known as the “hypotenuse” and the other two sides are known as the “legs.” That means, in trigonometry, the longest side of a right-angled triangle is still known as the “hypotenuse” but the other two legs are named to be:
- opposite side and
- adjacent side
We decide the “opposite” and “adjacent” sides based upon the angle which we are talking about.
- The “opposite side” or the perpendicular is the side that is just “opposite” to the angle.
- The “adjacent side” or the base is the side(other than the hypotenuse) that “touches” the angle.
Sin Cos Tan Values
Sin, Cos, and Tan values in trigonometry refer to the values of the respective trigonometric function for the given angle. We can find the sin, cos and tan values for a given right triangle by finding the required ratio of the sides. Let us understand the formulas to find these ratios in detail in the following sections.
Sin, cos, and tan functions in trigonometry are defined in terms of two of the three sides (opposite, adjacent, and hypotenuse) of a right-angled triangle. Here are the formulas of sin, cos, and tan.
sin θ = Opposite/Hypotenuse
cos θ = Adjacent/Hypotenuse
tan θ = Opposite/Adjacent
Apart from these three trigonometric ratios, we have another three ratios called csc, sec, and cot which are the reciprocals of sin, cos, and tan respectively. Let us understand these sin, cos, and tan formulas using the example given below.
Example: Find the sin, cos, and tan of the triangle for the given angle θ.
Solution:
In the triangle, the longest side (or) the side opposite to the right angle is the hypotenuse. The side opposite to θ is the opposite side or perpendicular. The side adjacent to θ is the adjacent side or base.
Now we find sin θ, cos θ, and tan θ using the above formulas:
sin θ = Opposite/Hypotenuse = 3/5
cos θ = Adjancent/Hypotenuse = 4/5
tan θ = Opposite/Adjacent = 3/4
Trick to remember sin cos tan formulas in trigonometry: Here is a trick to remember the formulas of sin, cos, and tan. We can use the acronym “SOHCAHTOA” as shown below,
The trigonometric ratios, sin, cos, and tan do not exactly depend upon the side lengths of the triangle but rather they depend upon the angle because ultimately, we are taking the ratio of the sides. Sin, cos, and tan table is used to find the value of these trigonometric functions for the standard angles. During calculations involving sine, cosine, or tangent ratios, we can directly refer to the trig chart given in the following section to make the deductions easier.
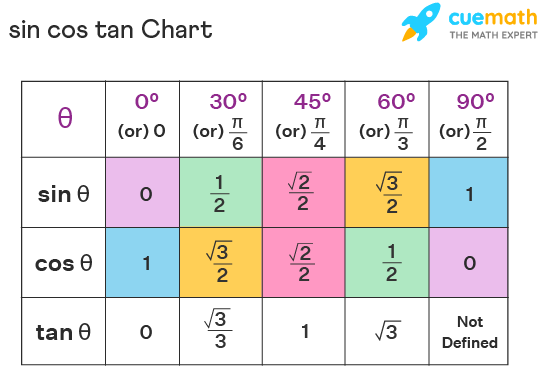
Sin Cos Tan Chart
Sin cos tan chart/table is a chart with the trigonometric values of sine, cosine, and tangent functions for some standard angles 0o, 30o, 45o, 60o, and 90o. We can refer to the trig table given below to directly pick values of sin, cos, and tan values for standard angles.
The tips that you need to memorize from this chart are:
- The angles 0o, 30o, 45o, 60o, and 90o in order.
- The first row (of sin) can be remembered like this: 0/2, √1/2, √2/2, √3/2.
- That’s all you need to remember because: The row of cos is as same as the row of sin just in the reverse order.
- Each value in the row of tan is obtained by dividing the corresponding values of sin by cos because tan = sin/cos.
You can see how is tan = sin/cos here:
sin θ/cos θ = (Opposite/Hypotenuse) ÷ (Adjacent/Hypotenuse) = (Opposite/Hypotenuse) × (Hypotenuse/Adjacent) = Opposite/Adjacent = tan θ
The values of sin, cos, and tan can be calculated for any given angle using the unit circle. Unit circle in a coordinate plane is a circle of unit radius of 1, frequently centered at the origin (0, 0) in the Cartesian coordinate system in the Euclidean plane, especially in trigonometry. For any point on unit circle, given with the coordinates(x, y), the sin, cos and tan ratios can be given as,
- sin θ = y/1
- cos θ = x/1
- tan θ = y/x
where θ is the angle the line joining the point and origin forms with the positive x-axis.
Trigonometry ratios sin, cos, tan find application in finding heights and distances in our daily lives. We use sin, cos, and tan to solve many real-life problems. Here is an example to understand the applications of sin, cos and tan.
Example: A ladder leans against a brick wall making an angle of 50o with the horizontal. If the ladder is at a distance of 10 ft from the wall, then up to what height of the wall the ladder reaches?
Solution:
Let us assume that the ladder reaches till x ft of the wall.
Using the given information:
Here, we know the adjacent side (which is 10 ft) and we have to find the opposite side (which is x ft). So we use the relation between the opposite and the adjacent sides which is tan.
tan 50o = x/10
x = 10 tan 50o
x ≈ 11.9 ft
Here, tan 50o is calculated using the calculator and the final answer is rounded up to 1 decimal. Therefore, the ladder reaches up to 11.9 ft of the wall.
Topics Related to Sin Cos Tan:
- Sine Law
- Cosine Law
- What is a Radian
- Trigonometric Ratios in Radians
- Tangent Function
- Heights and Distances